Neu Mass & Charge Radii
of
Natural & Selected Radioactive Isotopes
Introduction
The Neu Mass & Charge Radii calculates the nuclear physical properties of approximately 350 natural and selected radioactive isotopes based on a topological model that uses a hypothesized invariant 3 part neutron structure as a natural quantum unit of atomic matter. See Figure 0.4 – The Primal Prototype Object.
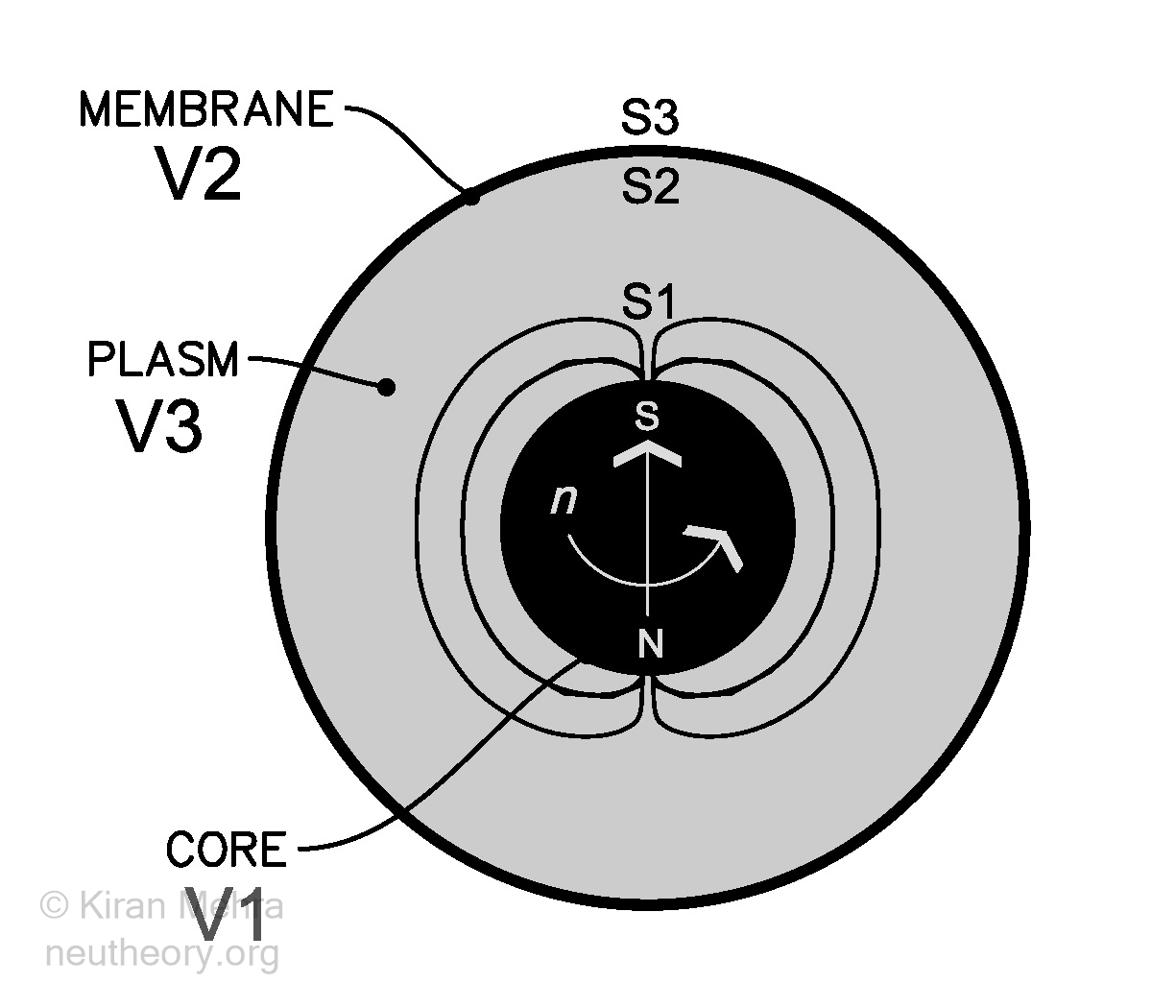Figure 0.4 – The Primal Prototype ObjectThe currently accepted atomic mass unit (amu) is the Dalton unified atomic mass unit which is based on 1/12th of the mass of a C12 atom, and is calculated as approximately equal to 1.660 539 066 x 10-27 kg. With this NIST standard the relative mass of a neutron is equal to 1.008 664 915 amu.
Neu Theory in principle sets the neutron equal to one atomic matter unit. The Neu Theory amu is called the neu (neutron equivalent unit) and its measured value is approximately equal to 1.674 927 498 x 10-27 kg of mass. The neu is an absolute unit – a precise invariant quantity – numerically equal to exactly one quantum unit of matter in nature.
The neu is slightly larger than the C12 amu representing an increase in relative mass value of approximately 0.8665 %. The atomic masses of the isotopes in the table are then compared to the neu unit quantity with a corresponding decrease in relative mass value of approximately 0.8595 % as compared to the C12 value. The absolute neu value of C12 becomes 11.896 914 249 instead of 12.000 000 000. Column I in the table provides the relative C12 values as published by the National Institute of Science and Technology (updated September 21, 2016), Column J provides the equivalent calculated absolute neu mass value of selected isotopes.
It should be clearly emphasized that the actual measured mass of atoms in kilograms and the equivalent energy value in joules, electron volts, or any other unit, is not changed in the slightest by using a neutron amu. The numerically smaller quantum neu mass value of an isotope is exactly balanced by the numerically larger quantum neu mass unit giving us precisely the same quantity of mass expressed in kilograms, that we had before.
The reason an absolute neu can be useful, is that it allows mass and energy to be counted with the same numeric scale, and allows us in principle to estimate the ratio of matter to energy in the cosmos.
The table consists of two major parts:
- Columns A-Z – Neu Mass and other derived atomic values
- Columns AA-BL – Charge Radius derived values
All nuclides have an effective physical volume that is measured by their charge radius, a quantity that can be precisely measured, and is capable of providing a clear difference between the relative size of isotopes. The Neu Theory charge radii table uses values empirically determined and originally published in September 1999 by the International Nuclear Data Committee of the International Atomic Energy Agency and the adjusted values published in December 2012 by I. Angeli and K.P. Marinova. Some charge radii of some radioactive isotopes are not available. If these source values change or additional charge radii values become available, the table values will correspondingly adjust.
For simplicity, the charge radius is considered as defining the limiting spheroidal volume within which the neucleon cluster fits. In reality it is hypothesized, the charge shield will uniformly cover the underling cluster, whatever its shape. The neucleon membrane walls do a “best fit”, and the neuclonic plasms adjust their density (volume) as needed until a stable cluster configuration is reached.
Nuclear Particles
The Neu theory nuclear model uses 5 of the 7 elementary particles of nature [1a][2a][3a][1b][6+]. These are the only physical forms that play an active role in all nuclear structure.
| tbl col | form # | elementary particle | shape | substance | neu value | volume | density | thickness | structure | spin/magnetism | g-force |
| O | [1a] | neutron core | sphere | *Type I matter | 0.998623u | fixed | fixed | (= r) | homogenous | ↑↓ = -1.000 | g-rise |
| P | [2a] | neutron membrane | deformable shell | *Type I matter | 0.000544u | fixed | fixed | varies | homogenous | none | g-rise |
| Q | [3a] | neutron plasm | deformable shell | **Type II matter | 0.000833u | varies | varies | varies | homogenous | none | g-rise |
| S | [1b] | captive proton core | sphere | *Type I matter | ≤0.996255u | varies | fixed | (= r) | homogenous | ↑↑ = (+ value varies with size) | g-rise |
| AD | [6+] | positive charge shell | deformable shell | split spin energy | (½ 0.000833u) | fixed | fixed | varies | homogenous | none | g-fall |
*Type I matter is at an absolute density of ~6.693 x 1017 kg/m3, and a specific volume of ~2.502 x 10-45 m3/neu.
**Type II matter varies in volume & density with nuclides.
These 5 particles make the three neucleons, which make all the nuclides that are listed as the elements and isotopes in the table. For each isotope the table generates fundamental properties at three subatomic levels:
- The 3rd or ordinary level is the measured and calculated physical properties of the isotope nucleus as a whole, i.e., neucleon makeup, total neu mass, net spin/magnetism, charge radius, nuclide volume, average density, nuclide g-rise, average electric charge shell thickness.
- The 2nd or primary level is the physical properties of the individual neucleon cells, i.e., cell neu mass, average volume , average density of the cells that make up the isotope.
- The 1st or elementary level (nature’s “bottom”) are the individual elementary particles, i.e., the calculated physical properties of the individual cores, membranes, plasms and charge shells that make up the different elements and isotopes.
Neucleon Cells
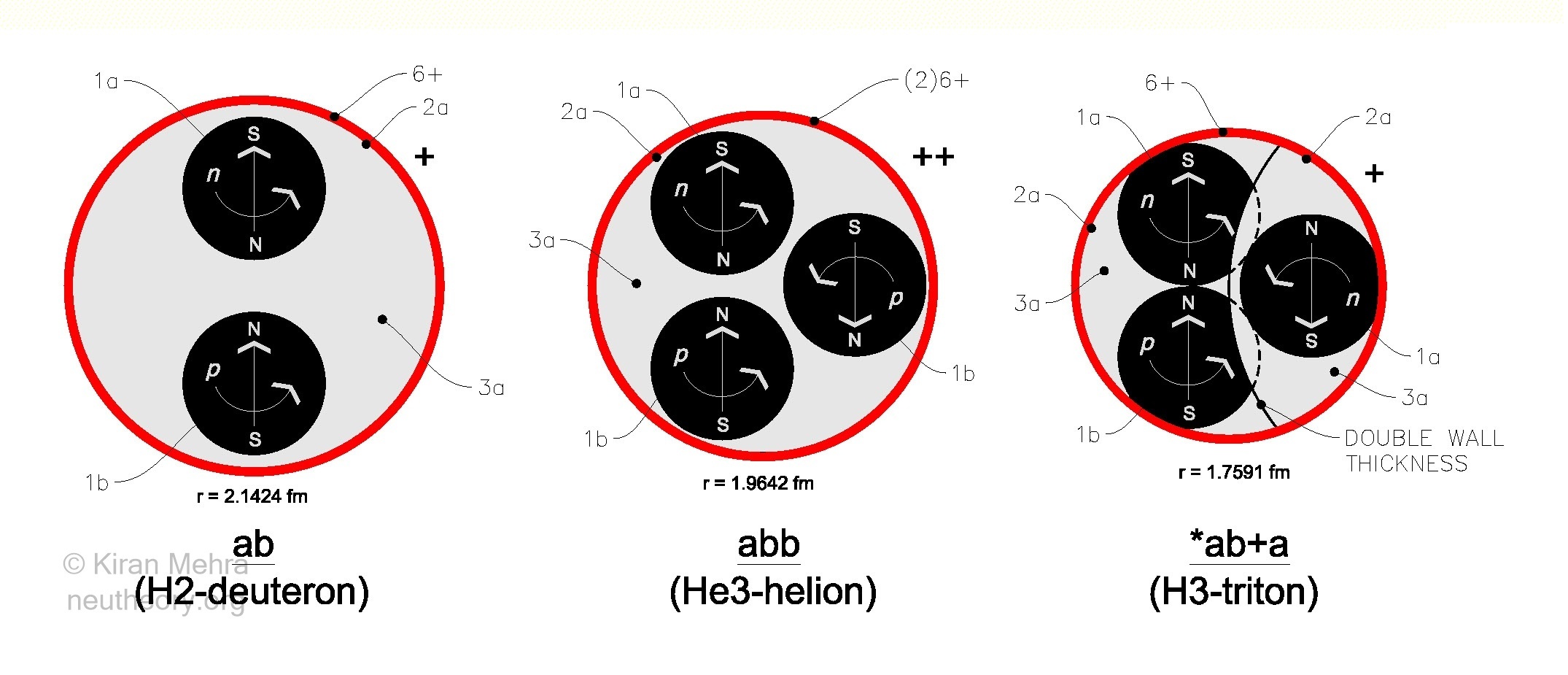Figure 4.4 – The Three Neucleons
All atomic nuclides in nature are built of some combination of three primary cells called neucleons. See Figure 4.4 – The Three Neucleons. The size and structure of the 3 free neucleons is as noted and they are drawn to approximate relative scale. Neutron membrane thickness [2a] and charge shell thickness [6+] is symbolic and not to scale.
- The ab deuterons (Column F) are built from 5 elementary particles [1a][2a][3a][1b][6+]
- The abb helions (no column provided in the table) are built from 7 elementary particles [1a][2a][3a][1b][1b][6+][6+]
- The a neutrons (Column G) are built from 3 elementary particles [1a][2a][3a] and as they have no charge shell of their own, are only stable below some other nuclide charge shield. Hydrogen-3 with 1 deuteron + 1 neutron is shown (*ab+a).
In this model all stable nuclides and most radioactive nuclides are made from deuterons and neutrons only. The helion (He3) is stable as a free nuclide, however is unstable as a neucleon within a larger cluster, radioactively transforming (typically by positron emission) until a stable nuclide cluster is reached that contains only deuteron and neutron cells.
All nuclide clusters are held together below concentric layers of positive electric charge shells equal to the deuteron number. It should be noted that the model requires that there be no individual proton surfaces with an attached charge shell within a nuclide.
All protons within a nuclide are captive protons within neutron cells with their electric charge shells having migrated above the neutral neutron membrane surface(s). It is hypothesized by the model, that all nuclide mass loss (or gain) from radioactivity, comes from captive protons only. This reduction of proton mass is considered as the “binding mass” loss required to maintain nuclear stability, and in this model, is equivalent to twice the “binding energy” value of conventional science.
Neu Theory does not require a “strong” attractive nuclear force to hold the nucleus together. Uniform universal acceleration of the electric charge shield envelope creates g-fall, a compression force that acts in an opposite direction to nuclide cluster g-rise (AH). The reaction to g-rise pressure creates tension within the charge shield, and its thickness (AC) decreases. When equilibrium is reached between g-rise (AH) pressure and g-fall tension (in principle, the charge compression value is equal and opposite to g-rise), a stable nuclear size and stable average charge shell thickness (AD) is maintained.
Table Layout
| Column | Column Title | Description |
| A | Symbol | Chemical abbreviation for element. |
| B | Atomic name | Atomic name of element. |
| C | Natural abundance | Isotopic abundance as found in nature in percent. Radioactive isotopes are indicated by *. |
| D | Core # | Number of cores in the nuclide. Core number is the same as the SI mass number “A”. |
| E | Neucleon # | Number of neucleon cells contained within the nuclide charge shield. This is the cluster number “C” |
| F | Deuteron # | Number of deuterons (ab-state) in the nuclide. This is the same as the SI atomic number “Z”, and the nuclear charge shield number. |
| G | Neutron # | The number of neutron neucleons (a-state) in the nucleus. |
| H | Electron # | Total number of electrons in the neutral atom. This is the same as the atomic number Z. |
| I | C-12 atomic mass unit | Lists values for atomic mass relative to the mass of the Carbon 12 isotope which has been assigned a value of 12.000000 atomic mass units. |
| J | Neu atomic mass unit | Lists values for atomic mass of nuclides relative to the mass of one neutron which has been assigned an absolute value of 1.000000 atomic mass units. These values are derived by dividing C12 values by 1.008665. |
| K | Neu Energy | The difference between the core (mass) number and neu atomic mass of the atom (D-J). This measures the absolute value of de-linked energy from the atom. |
| L | Electron mass | The total value of the atomic neu mass carried by electrons. |
| M | Nuclide mass | The total value of atomic neu mass carried by the nuclide (J-L). |
| N | Neutron mass | The total value of neutron mass carried by the nuclide. This is always an integer value equal to the neucleon number (E). |
| O | Neutron Core mass | The total value of neu mass carried by the neutron cores of the nuclide (E x 0.998623). |
| P | Neutron Membrane mass | The total value of neu mass carried by the neutron membranes of the nuclide (E x 0.000544). |
| Q | Neutron Plasm mass | The total value of neu mass carried by the neutron plasms of the nuclide (E x 0.000833). |
| R | Proton Core mass | The total value of neu mass carried by protons (captive to deuterons and helions) in the nuclide. This value is derived by subtracting the neutron and electron mass from the nuclide mass (J-N-L). |
| S | Mass per proton | The total value of proton neu mass divided by the number of protons (R/F). |
| T | Mass loss per proton | The reduced neu mass value of each proton in the nucleus. This measures the absolute value of de-linked spin & rise energy from each proton (0.998623-S). |
| U | Neucleons | The number of each type of neucleon contained by the nuclide: 2s = deuterons (net spin 2 up • ↑↑); 3s = helions (net spin 1 up • ↑↑↓); 1s = neutrons (net spin 1 up • ↑). Note: It is the net spin of each neucleon that contributes to the net spin/magnetism of a nuclide. |
| V | Neu spin | The value and orientation of net residual nuclear spin after neucleonic alignment of the cores. The neu spin values are derived by multiplying the current (h/2π) one-half integer values by 2, changing them into integer values. The orientation of the neu spin vector does not change. The neu spin values of the nucleons are: deuteron = 2 up (↑↑); helion = 1 up (↑↑↓); neutron = 1 up (↑). |
| W | Neu magneton | The value of the nuclear magnetic moment relative to the magnetic moment of the neutron which has been assigned an absolute value of -1.0000 magneton (anti-parallel). The values are derived by dividing nuclear magnetic moment values by 1.9131. The north/south magnetic dipole orientation does not change. |
| X | Half-life | Half-life of a radioactive nuclide: s = seconds, m = minutes, d = days, y = years. |
| Y | Decay mode | Radioactive decay symbols: α = alpha emission, β- = electron emission, β+ = positron emission, E.C. = electron capture. Gamma emission is not included in this table. |
| Z | Decay Products | Daughter isotope(s) remaining after transition. |
| AA | Charge radius | The charge radius of the nuclide in femtometer or fermi units (10-15 m). The table uses values empirically determined and originally published in September 1999 by the International Nuclear Data Committee of the International Atomic Energy Agency and the adjusted values published in December 2012 by I. Angeli and K.P. Marinova. Neu Theory considers this value as one/half the average inside diameter of the charge shell surrounding the nuclide cluster membrane. The thickness of the charge shield is measured from this radius. |
| AB | Electric Quadrupole | Electric quadrupole in barns (10-28/m2). A positive value suggests a prolate (cigar shape) nuclide around the spin axis A negative value suggests an oblate (disk shape) nuclide around the spin axis. A zero value indicates a spherical shape around the spin axis. The quadrupole values of many isotopes are not available. |
| AC | Charge Shield “t” | Estimated minimum thickness of the total nuclide charge shield in 10-20 m units. Estimate is based on the starting assumption that the spherical charge shield spin energy has a specific volume equal to the specific volume of Type I spinrise. |
| AD | Avg. Charge Shell “t” | Average thickness of each charge shell in 10-20 m units. Thickness is calculated by dividing the total charge shield thickness by the number of charge shells (AC/F). |
| AE | Avg. Nuclide diameter | The nuclide diameter in fermi’s (fm, 10-15 m). The charge radius multiplied by 2 (AAx2). |
| AF | Nuclide volume | Nuclide volume in units of cubic fm (10-45 m3). |
| AG | Nuclide density | Nuclide density in units of 1017 kg/m3. |
| AH | Nuclide g-rise | Nuclide g-rise in units of 10-7 m/s2. Acceleration due to Rise – g = Gm/r2 , G = 6.67384×10-11 newton meters squared per kilogram squared (N x m 2 x kg –2 ) |
| AI | Core volume | Total volume of core Type I matter in cubic fm (10-45 m3). Volume is calculated by multiplying total core neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu (M-P-Q x 2.5025). |
| AJ | Membrane volume | Total volume of membrane Type I matter in cubic fm (10-45 m3). Volume is calculated by multiplying total membrane neu mass by the specific volume of spinrise @ 2.502 x 10-45 m3/neu (P x 2.5025). |
| AK | Plasm volume | Total volume of plasm Type II matter in cubic fm (10-45 m3). Volume is calculated by subtracting total core and membrane volume from the nuclide volume (AF-AI-AJ). |
| AL | Plasm density | Plasm density in units of 1014 kg/m3. Density is calculated by dividing the total plasm neu mass divided by the total plasm volume (Q/AK). |
| AM | Plasm density % max | Nuclide plasm density as a percentage of maximum density (AL/6.693034 x 100). |
| AN | Avg. Plasm volume | Total plasm volume divided by the neucleon number (AK/E). |
| AO | Total ab volume | Average ab volume multiplied by the deuteron number (F x AP). |
| AP | Avg. ab volume | Volume of average deuteron in the nuclide. Volume is calculated by multiplying total core and membrane neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu, and adding the average plasm volume ((0.999167+S) x 2.5025) +AN). |
| AQ | Plasm volume/%ab | Volume of the deuteron plasm in a nuclide as a percentage of the average deuteron volume (AN/AP x 100). |
| AR | Avg. ab density | Average density of a deuteron in a nuclide in units of 1017 kg/m3. The density is calculated by adding the neu mass of one neutron with the average neu mass of the captive proton and dividing by the average deuteron volume (1.0+S x 1.674929) / AP). |
| AS | Avg. ab diameter | The average ab diameter of a nuclide deuteron in fm units (10-15) is equal to the diameter of a simple ball with an equivalent volume. The calculation is made using spherical geometry. |
| AT | Avg. ab plasm “t” | The average thickness of the plasm surrounding 2 cores of a nuclide deuteron in fm units (10-15). This is a topological simplification as plasm thickness varies within a neucleon. |
| AU | Plasm “t”/% core | The average plasm thickness as a percentage of the core radius (AT/0.84184 x 100). |
| AV | Captive p volume | The captive proton volume of a nuclide deuteron in cubic fm units (10-45 m3) is calculated by multiplying the captive proton neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu (S x 2.5025). |
| AW | Captive p diameter | The captive proton diameter of a nuclide deuteron in fm units (10-15 m) is calculated from the volume using spherical geometry. |
| AX | Proton qs cycle | The quantum spin cycle of a captive proton of a nuclide deuteron in units of 10-23 seconds. The time of one cycle (one revolution) is calculated by dividing the circumference of the proton by the speed of light. |
AY | Proton qs frequency | The quantum spin frequency of a captive proton of a nuclide deuteron in units of 1022 revolutions per second (1/AX). |
| AZ | Max. ab membrane “t” | The maximum membrane thickness of a nuclide deuteron in units of 10-20 m. The thickness is made from a fixed volume of Type I matter in a shell with the surface area of the average deuteron. |
| BA | Total a volume | Average a volume multiplied by the neutron number (G x BB). |
| BB | Avg. a volume | Volume of average neutron in the nuclide. Volume is calculated by multiplying core and membrane mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu, and adding the average plasm volume ((0.999167 x 2.5025) +AN). |
| BC | Plasm volume/% a | Volume of the neutron plasm in a nuclide as a percentage of the average neutron volume (AN/BB x 100). |
| BD | Avg. a density | Average density of a neutron in a nuclide in units of 1017 kg/m3. The density is calculated by dividing the mass of one neutron by the average neutron volume (1.674929 / BB). |
| BE | Avg. a diameter | The average diameter of a nuclide neutron in fm units (10-15) is equal to the diameter of a simple ball with an equivalent volume. The calculation is made using spherical geometry. |
| BF | Avg. a plasm “t” | The average thickness of the neucleonic plasm surrounding the core of a nuclide neutron in fm units (10-15). This is a topological simplification as plasm thickness varies within a neucleon. |
| BG | Max. a membrane “t” | The maximum membrane thickness of a nuclide neutron in units of 10-20 m. The thickness is made from a fixed volume of Type I matter in a shell with the surface area of the average neutron. |
| BH | Nuclide packing % | The nuclide packing density as a percentage of maximum density. This is calculated by multiplying the nuclide neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu and dividing by the nuclide volume ((M x 2.5025) /AF x100). |
| BI | ab cell packing % | The deuteron packing density as a percentage of maximum density. This is calculated by multiplying the deuteron neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu and dividing by the average deuteron volume ((1.0+S) x 2.5025) /AP x100). |
| BJ | a cell packing % | The neutron packing density as a percentage of maximum density. This is calculated by multiplying the neutron neu mass by the specific volume of spinrise @ 2.5025 x 10-45 m3/neu and dividing by the average neutron volume (1.0 x 2.5025/BB x100). |
| BK | Atomic radius | Atomic radius as computed in pm (10-12 m). Electrons are contained within this volume. Not isotope specific. Accepted values. |
| BL | Van Der Waals radius | Van Der Waals radius in pm (10-12 m) defines half of the distance between the closest approach of two non-bonded atoms of a given element. In Neu Theory this distance is the height of the spinfield hollow of atoms. Not isotope specific. Accepted values. |
ORIGINAL DATA SOURCE
Columns A, C, D, V, W, X, Y, Z – Handbook of Chemistry and Physics, 1988 edition, by CRC Press, Inc.: Wikipedia
Column I – Atomic Weights and Isotopic Compositions, National Institute of Science and Technology, September 2016
Column AA – Table of Experimental Nuclear Ground State Charge Radii: An Update, By I. Angeli and K.P. Marinova, December 2012
Column AB – Nuclear Isotope Database, EasySpin.org; (Pyykko and others)
Neu Mass & Charge Radii Table
(click on image to go to table)
